The Arbelos
... and a New Relationship

by

 Markus Heisss

 Würzburg, Bavaria

  05/2022 (Last update: August 1, 2022)

    The copying of the following graphics is allowed, but without changes.

[To get a bigger picture, please click it with the cursor.]

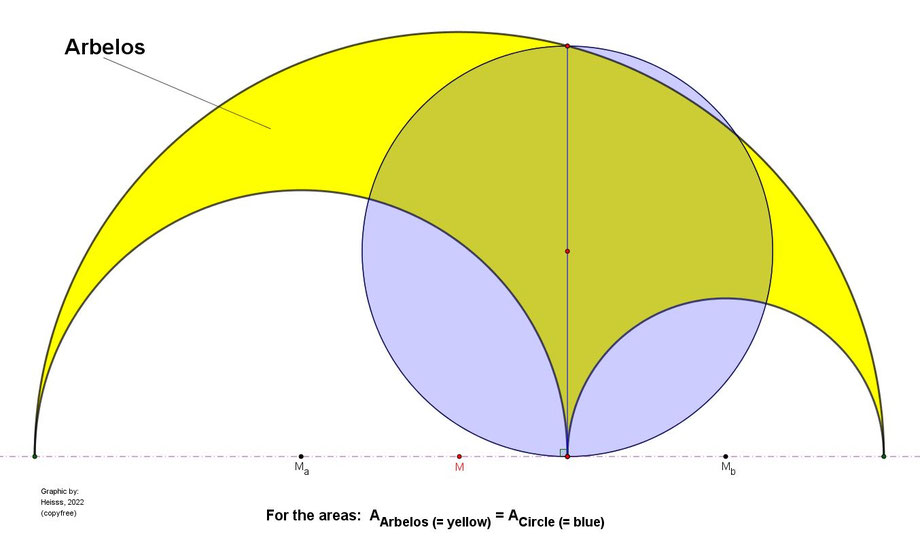
The important information you'll find in the graphics.

 

 

Arbelos, shoemaker's knife, area, geometry, Markus Heisss

 

Arbelos (- Greek: ἄρβυλος [árbylos] -) means "shoemaker's knife",

because the geometrical figure resembles the blade of such a knife.

 

  

Further information you can find at websites

like 'Wikipedia' [here]

or 'Wolfram MathWorld' [here].

What you WON'T find at these important sources are the following relationships:

 

Arbelos, geometry, tangent, Markus Heisss

 

And another relationship at the Arbelos:

 

Arbelos, circles, tangents, theorem,

 

The circle at point D with line segment DB as radius

contains the following relationship of a cyclic hexagon with only one axis of symmetry:

 

hexagon, cyclic, chords, one axis of symmetry

 

*********************************

 

Sometimes the construction of the incircle of the Arbelos is asked.

 

This isn't so easy as it seems.

One of several methods is shown in the following graphic:

 

 

Arbelos, incircle, construction, Heisss

 

Cite this as: 

Heisss, Markus:  From: https://triangle-geometry.jimdofree.com/arbelos/


*****************************

 

Are you interested in my other geometrical discoveries?

[here]

Contact

... to the author is maybe possible via e-mail under:   §@gmx.de   where   § = heisss

Subject: "Arbelos"