Frégier's Theorem
... and Several Discoveries

by

Markus Heisss

Würzburg, Bavaria

05/2020 (Last update: March 10, 2021)

 

  The copying of the following graphics is allowed, but without changes.

  [To get a bigger picture, please click it with the cursor.]

 

The first drawing shows Frégier's Theorem:

 

Frégier Fregier point, ellipse, conic section, hyperbola, parabola, right angle
Fig. 01: Frégier's Theorem

 

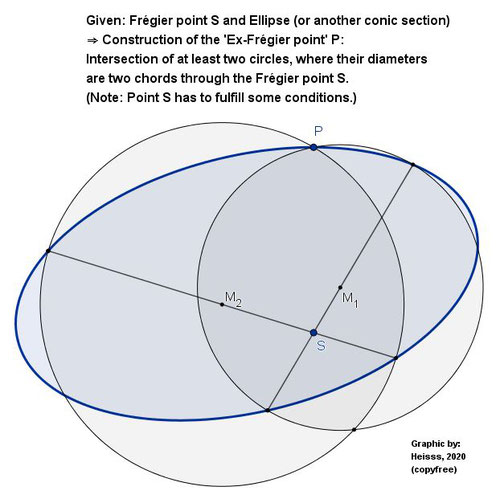
As next the reverse case:

The Frégier point S is given

and point P on the curve shall be constructed:

 

Frégier's theorem, Fregier, ellipse, conic section, geometry, science, Würzburg, Germany
Fig. 02: Construction of the Ex-Frégier Point

 

This starting point P has---so far as I know---no official designation yet.

Therefore in the further course it will be named "Ex-Frégier point".

(More on that further below.)

 

Now diverse conic sections can be examined,

on which important points lie.

 

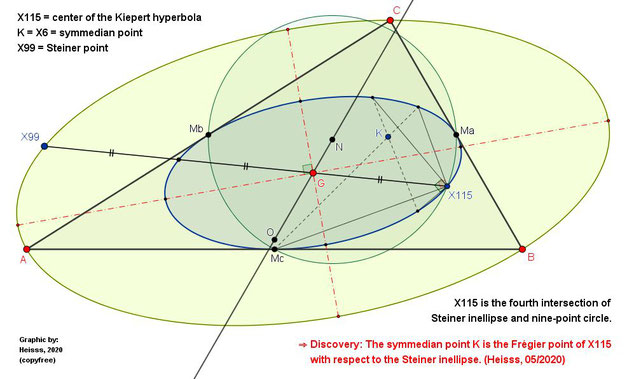
So lies for example the center of the Kiepert hyperbola on the Steiner inellipse.

See next graphic:

 

Frégier point, Fregier, symmedian point, Steiner point, inellipse, circumellipse, conic section
Fig. 03: Frégier's Theorem; Center of the Kiepert Hyperbola X115 wrt Steiner Inellipse

 

It shows up, that the symmedian point K (=X6)

is the Frégier point of the center of the Kiepert hyperbola (=X115)

with respect to the Steiner inellipse.

Or reversely said:

Is the Frégier point the symmedian point K with respect to the Steiner inellipse,

then the "Ex-Frégier point" is the center of the Kiepert hyperbola (=X115).

 

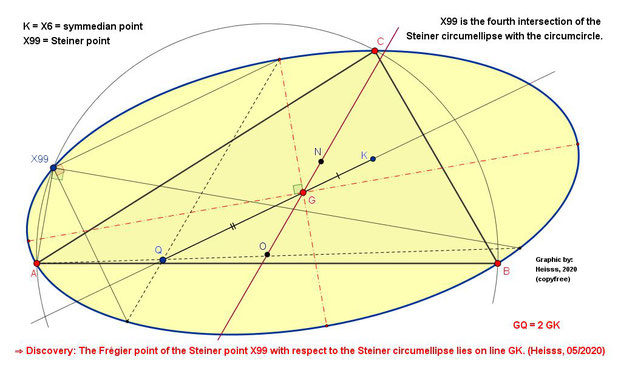
Now analogue with the Steiner point (=X99)

with respect to the Steiner circumellipse:

 

Frégier point, Heisss, Fregier, geometry, geometrical discovery
Fig. 04: Frégier's Theorem; Steiner Point wrt Steiner Circumellipse

 

Unfortunately, the Frégier point Q of the Steiner point X99

with respect to the Steiner circumellipse

is not an important center of triangle ABC.

 

 

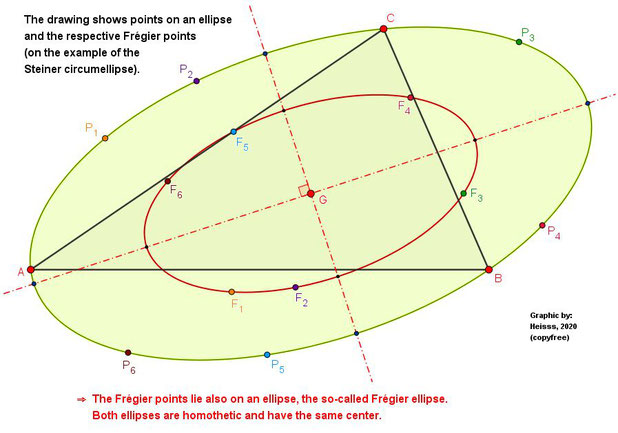
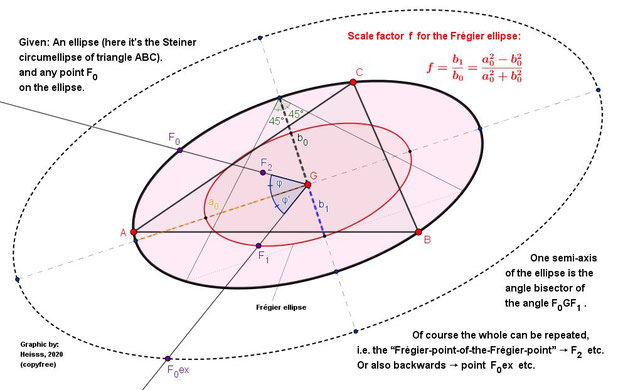
The next graphic shows the Frégier points of several different points,

which lie all on the same conic section:

 

Frégier ellipse, Fregier, Frégier point, Frégier's theorem, Steiner circumellipse
Fig. 05: Frégier Ellipse as Example of the Steiner Circumellipse

 

The Frégier points of the point of an ellipse lie also on an ellipse,

a so-called "Frégier ellipse".

 

The next graphic shows an interesting case:

The "Frégier-point-of-the-Frégier-point"

with respect to the Frégier ellipse:

 
Fregier ellipse, Frégier point, Steiner circumellipse, Heisss
Fig. 06: Scale Factor of the Frégier Ellipse

 

This knowledge could be used for the construction of the semi-axes of the Steiner circumellipse,

because the axes are the respective angle bisectors of angles KGX99 and KGX115.
But there is a faster method for constructing the axes of the Steiner circumellipse. [here]

 

Postscript (March 10, 2021):

D. Reznik & M. Helman made further investigations on this phenomenon

and found some other relationships which can be seen in a dynamic graphic video on YouTube.

Really recommendable!

Would you like to see it?

Than click [here].

 


*************

 

 

In the analogue case of a parabola or hyperbola

you'll get a "Frégier parabola" or a "Frégier hyperbola".

 

But:

The Kiepert-Hyperbel is a special case!

On the Kiepert hyperbola lie

several importent Kimberling centers lie on it, as ...

centroid G (=X2), orthocenter H (=X4), X10 (= Spieker point),

X13 (= first Fermat point), X14 (= second Fermat point),

X17 (= first Napoleon point), X18 (= second Napoleon point),

X98 (= Tarry point), etc.

 

[A table of the so-called Kimberling centers

you'll find here.]

 

Now a graphic, which shows the Kiepert hyperbola:

 

center of the Kiepert hyperbola, Napoleon point, Fermat point
Fig. 07: Kiepert Hyperbola

 

[Further information on the Kiepert hyperbola

you can find here.]

 

Now the Frégier point of centroid G with respect to the Kiepert hyperbola:

 

Frégier point, point at infinity
Fig. 08: Frégier's Theorem; Centroid X2 wrt Kiepert Hyperbola

 

It shows up, that in this case

the Frégier point has to be a point at infinity.

 

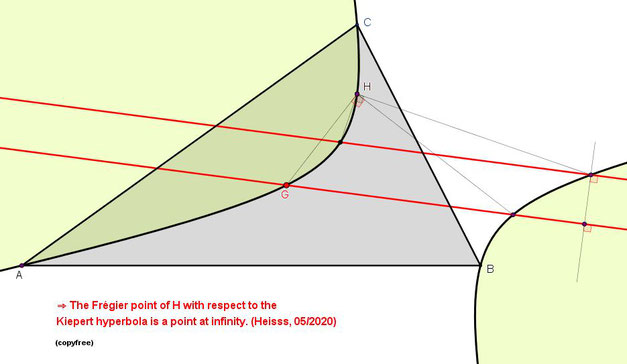
Similarly the Frégier point of orthocenter H with respect to the Kiepert hyperbola:

 

by Markus Heisss, Würzburg, math, geometry, science not at the university
Fig. 09: Frégier's Theorem; Orthocenter X4 wrt Kiepert Hyperbola

 

The Frégier point is also a point at infinity.

 

Next trial with Tarry point (=X98) with respect to the Kiepert hyperbola:

 

Tarry point, Frégier point, point at infinity, parallel lines, Markus Heisss
Fig. 10: Frégier's Theorem; Tarry Point X98 wrt Kiepert Hyperbola

 

Again the Frégier point is a point at infinity.

 

In the three previous cases I made no further attempts to proove,

if these points at infinity are any Kimberling centers.

But:

Tests showed that every Frégier point of any point on the Kiepert hyperbola

with respect to the Kiepert hyperbola is a point at infinity.

 

Final Remark:

Maybe other conic sections with respect to the Frégier points are of interest,

like Brocard ellipse, Mandart ellipse, Kiepert parabola etc.

Or: Take important Kimberling centers as Frégier points

and determine the Ex-Frégier points.

Interesting connections are waiting!

 

Good luck with searching!

  

Cite this as: 

Heisss, Markus: From: https://triangle-geometry.jimdofree.com


*****************************

 

Are you interested in my other geometrical discoveries?

[here]

Contact

... to the author is maybe possible via e-mail under:   §@gmx.de   where   § = heisss

Subject: "Frégier's Theorem"